
Clemency Montelle: The (a)symmetry of a sari
Speaker: Prof Clemency Montelle
Affiliation: University of Canterbury
As they say mathematics is all around us. Therefore it should come as no surprise that you might be wearing some! This talk will be a light-hearted look at the ways in which clothing can epitomise various mathematical topics. From the humble t-shirt to the elaborate draping of a sari, we will explore some of the more abstract properties of outfits from around the world.
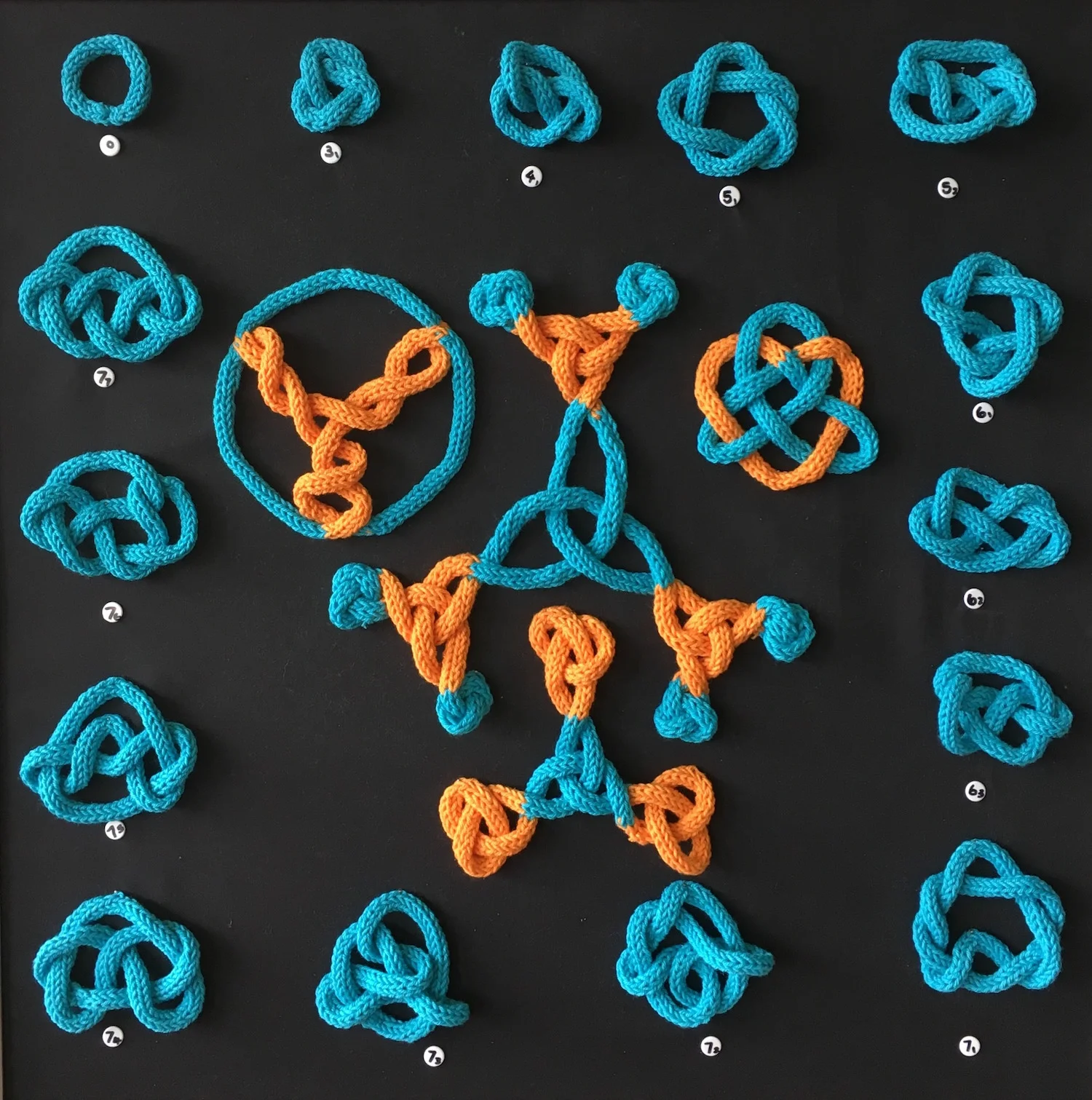
Ms Elizabeth Chesney: Knuts about Knitting Knots
Speaker: Ms Elizabeth Chesney
Affiliation: University of Canterbury
Knots have been used for thousands of years, with the earliest examples found dating back to prehistoric times. Besides their obvious function of tying objects together, they have been used for everything from recording information, to decoration and spiritual symbolism. Mathematicians are interested in knots for other reasons and study properties of knots related to their geometry in an area of mathematics known as knot theory. In this talk we’ll reveal some of the beauty and complexity of this subject using some simple knitted knots. We’ll see that while knitting is not knotting, we can certainly knit our way into knot theory!

Burkard Polster: What is the best way to lace your shoes?
Speaker: Burkard Polster
Affiliation: Monash University
What is the best way to lace your shoes? All the mysteries of shoelaces finally revealed! Find out about the shortest, strongest and silliest ways to lace your shoes, why your shoelaces come undone all the time and what all this has to do with mathematics, monkeys and salesmen. Don't miss this introduction to the secret life of shoelaces by the world's leading shoelace charmer.
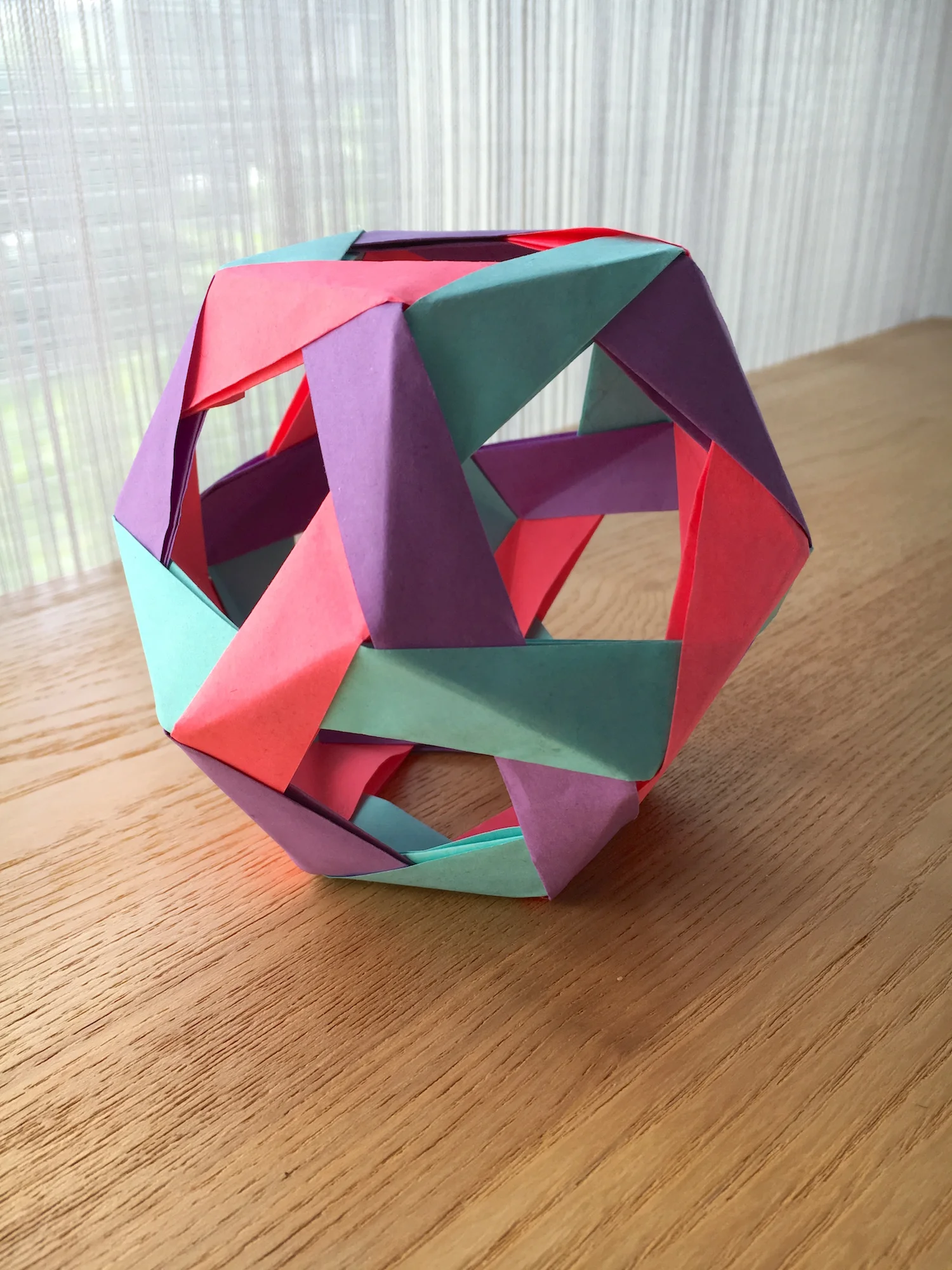
Dr Michael Assis: The beauty of origami / The beauty of mathematics -- connecting folds
Speaker: Dr Michael Assis
Affiliation: University of Melbourne
Inherent in a single piece of paper is a world of beauty and mathematics. The ancient paper folding art of origami has in recent decades seen an artistic and mathematical renaissance. From simple cranes and star puffs to complex tessellations and dragons, a survey of different areas of mathematics is needed to understand the folding properties of each model. The talk will walk through well known as well as new results in folding algorithms, origami software, and deep but beautiful mathematics, and along the way we will be discussing the impact this understanding has had on the art itself.

Jeanette McLeod: Four Colours are Enough
Speaker: Dr Jeanette McLeod
Affiliation: University of Canterbury
How many colours are needed to colour a map so that no two regions sharing a border are coloured with the same colour? What if you had to use the fewest number of colours possible? Mathematicians took over 100 years to find the answer to this question, and the result is one of the most controversial theorems of the 20th century! In this talk we will discuss the history of the infamous Four Colour Theorem, explain why mathematicians have struggled with it so much, and explore its links with crafts such as knitting, quilting, and colouring.

Michael Langton: Zigzags, Fractals, and Pleating
Speaker: Dr Michael Langton
Affiliation: University of Canterbury
Pleating fabric is a mathematically fascinating and historically fashionable craft involving folded patterns that often look like zigzags. Everyone understands zigzags, but how do you make folding zigzags efficient? What happens if the zigs and zags don't alternate perfectly? What happens if you do infinitely many of them? How do you fold a two-dimensional zigzag? What even is a two-dimensional zigzag? How many different fold patterns are possible? Exploring the answers to these questions will take us through papercraft, fractals, and the mechanics of pleating fabric yourself.

Bernd Krauskopf and Hinke Osinga: Chaos in Crochet and Steel
Speakers: Prof Bernd Krauskopf and Prof Hinke Osinga
Affiliation: University of Auckland
Bernd Krauskopf and Hinke Osinga are both Professors in Applied Mathematics at the University of Auckland, and they study how chaotic behaviour arises and can be explained mathematically. Their research led them into unexpected directions of craft and art when they realised that they could turn their computer-generated images of the famous chaotic Lorenz weather model into a concrete model via crochet. The so-called Lorenz manifold embodies the underlying unpredictability in an intriguing and hands-on way. At over 25,000 stitches, the crocheted piece is about a metre wide and has been the subject of considerable media attention world wide. The amazing mathematical properties of the Lorenz manifold also caught the attention of artist Benjamin Storch, who turned it into a steel sculpture. Hinke and Bernd will highlight some of the underlying geometry and their journey from maths into craft and art.

Professor Graham Farr: Making Links and Breaking Codes: The Mathematics of Bill Tutte
Speaker: Professor Graham Farr
Affiliation: Faculty of Information Technology, Monash University
William (Bill) Tutte (1917-2002) became a research mathematician while still an undergraduate at Cambridge in the late 1930s, broke the toughest Nazi codes while at Bletchley Park in the Second World War, and became one of the greatest mathematicians of the 20th century. His wartime work sparked the secret construction of Colossus, one of the first-ever computers, and saved countless lives. After the war, he led the development of the mathematics of networks, known as Graph Theory. His work was usually inspired by pure curiosity or entertaining puzzles, but has been applied in domains as diverse as electrical circuits, statistical physics and information visualisation. This talk tells the story of Tutte's life and mathematics, drawing inspiration for craft projects on the way.
(Photo from www.metronews.ca)

Dr Patrick O'Sullivan: The Measure of All Things: Myths, Maths and Other Aspects of Greek Art
Speaker: Dr Patrick O'Sullivan
Affiliation: University of Canterbury
This illustrated talk focuses on how the Greeks incorporated numbers, ratios and proportions into their poetry, architecture and visual arts: from Homer’s great epic poem on Troy, the Iliad, to iconic buildings such as the Parthenon and sculpture from the ‘High Classical’ period (c. 450-400 BC). But the Greeks also constantly altered and extended such formulas to enhance the emotional and psychological impact of their art and poetry: humanity, no less than maths, was the measure. Examples of Greek artworks from the Logie Collection in the Teece Museum, which embody some of these concepts and how they engage the viewer, will also feature in this talk.

Eugenia Cheng: Beyond Infinity
Speaker: Eugenia Cheng, School of the Art Institute of Chicago
How big is the universe? How many numbers are there? And is infinity + 1 the same as 1 + infinity? Such questions occur to young children and our greatest minds. And they are all the same question: What is infinity? In this talk we will go on a staggering journey from elemental math to its loftiest abstractions. Along the way, we will consider how to make infinite music, how to take infinite selfies, how to create infinite cookies from a finite ball of dough, and how one little symbol holds the biggest idea of all.

Hinke Osinga: Chaos, Kayaking and Crochet
Speaker: Hinke Osinga
Affiliation: University of Auckland
Hinke Osinga is Professor in Applied Mathematics at the University of Auckland. Her research in dynamical systems theory became well known to the general public in 2004, when she turned her computer-generated images of chaotic behaviour into a crocheted model, called the crocheted Lorenz manifold. The Lorenz manifold is a surface with an intriguing geometry that signifies the unpredictability of chaotic behaviour; it arises from a set of mathematical equations that represent a much simplified model for weather prediction. At over 25,000 stitches, the crocheted piece is about a metre wide and has been the subject of considerable media attention world wide. After this talk, maybe you would like to crochet your own!

Bernd Krauskopf: From Maths to Art and Chaos to Steel
Speaker: Bernd Krauskopf
Affiliation: University of Auckland
One of the research interests of mathematician Bernd Krauskopf of the University of Auckland is how chaotic behaviour may arise and can be explained mathematically. With Hinke Osinga and other collaborators he has been working on the famous Lorenz system, where the chaotic behaviour is organised by an amazing surface: the Lorenz manifold. Imagine a pancake that grows and grows to fill the entire space without creasing or developing self-intersections. This amazing object has been turned into a steel sculpture in a collaboration with artist Benjamin Storch. Bernd will highlight some of the underlying geometry of the Lorenz manifold, including its curvature properties, and explain the process used to turn it into art.












